Mathematics in China

A brief outline of the history of Chinese mathematics

Primary sources are Mikami's The Development of Mathematics in China and Japan and Li Yan and Du Shiran's Chinese Mathematics, a Concise History. See the bibliography below.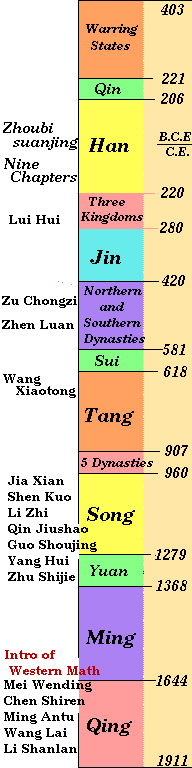

1. Numerical notation, arithmetical computations, counting rods
   
   
   
   • Traditional decimal notation -- one symbol for each of 1, 2, 3, 4, 5, 6, 7, 8, 9, 10, 100, 1000, and 10000. Ex. 2034 would be written with symbols for 2,1000,3,10,4, meaning 2 times 1000 plus 3 times 10 plus 4. Goes back to origins of Chinese writing.
     
   • Calculations performed using small bamboo counting rods. The positions of the rods gave a decimal place-value system, also written for long-term records. 0 digit was a space. Arranged left to right like Arabic numerals. Back to 400 B.C.E. or earlier.
     
   • Addition: the counting rods for the two numbers placed down, one number above the other. The digits added (merged) left to right with carries where needed. Subtraction similar.
     
   • Multiplication: multiplication table to 9 times 9 memorized. Long multiplication similar to ours with advantages due to physical rods. Long division analogous to current algorithms, but closer to "galley method."
     

Zhoubi suanjing (The Arithmetical Classic of the Gnomon and the Circular Paths of Heaven) (c. 100 B.C.E.-c. 100 C.E.)

• Describes one of the theories of the heavens. Early Han dynasty (206 B.C.E -220 C.E.) or earlier. Book burning of 213 B.C.E..
  
• States and uses the Pythagorean theorem for surveying, astronomy, etc. Proof of the Pythagorean theorem.
  
• Calculations including with common fractions.
  

The Nine Chapters on the Mathematical Art (Jiuzhang Suanshu) (c. 100 B.C.E.-50 C.E.)
Collects mathematics to beginning of Han dynasty. 246 problems in 9 chapters. Longest surviving and most influential Chinese math book. Many commentaries.

• Ch 1, Field measurement: systematic discussion of algorithms using counting rods for common fractions including alg. for GCD, LCM; areas of plane figures, square, rectangle, triangle, trapezoid, circle, circle segment, sphere segment, annulus -- some accurate, some approximations.
  
• Ch 2,3,6 on proportions, Cereals, Proportional distribution, Fair taxes.
  
• Ch 4, What width?: given area or volume find sides. Describes usual algorithms for square and cube roots but takes advantage of computations with counting rods
  
• Ch 5, Construction consultations: volumes of cube, rectangular parallelepiped, prism frustums, pyramid, triangular pyramid, tetrahedron, cylinder, cone, and conic frustum, sphere -- some approximations, some use pi=3
  
• Ch 7, Excess and deficients: false position and double false position
  
• Ch 8, Rectangular arrays: Gives elimination algorithm for solving systems of three or more simultaneous linear equations. Involves use of negative numbers (red reds for pos numbers, black for neg numbers). Rules for signed numbers.
  
• Ch 9, Right triangles: applications of Pythagorean theorem and similar triangles, solves quadratic equations with modification of square root algorithm, only equations of the form x^2 + a x = b, with a and b positive.
  

Sun Zi (c. 250? C.E.)
Wrote his mathematical manual. Includes "Chinese remainder problem" or "problem of the Master Sun": find n so that upon division by 3 you get a remainder of 2, upon division by 5 you get a remainder of 3, and upon division by 7 you get a remainder of 2. His solution: Take 140, 63, 30, add to get 233, subtract 210 to get 23.

Liu Hui (c. 263 C.E.)

• Commentary on the Nine Chapters
  Approximates pi by approximating circles polygons, doubling the number of sides to get better approximations. From 96 and 192 sided polygons, he approximates pi as 3.141014 and suggested 3.14 as a practical approx.
  States principle of exhaustion for circles
  Suggests Calvalieri's principle to find accurate volume of cylinder
  
• Haidao suanjing (Sea Island Mathematical Manual). Originally appendix to commentary on Ch 9 of the Nine Chapters. Includes nine surveying problems involving indirect observations.
  

Zhang Qiujian (c. 450?)
Wrote his mathematical manual. Includes formula for summing an arithmetic sequence. Also an undetermined system of two linear equations in three unknowns, the "hundred fowls problem"

Zu Chongzhi (429-500) Astronomer, mathematician, engineer.

• Collected together earlier astronomical writings. Made own astronomical observations. Recommended new calendar.
  
• Determined pi to 7 digits: 3.1415926. Recommended use 355/113 for close approx. and 22/7 for rough approx.
  
• With father carried out Liu Hui's suggestion for volume of sphere to get accurate formula for volume of a sphere.
  

Liu Zhuo (544-610) Astronomer
Introduced quadratic interpolation (second order difference method).

Wang Xiaotong (fl. 625) Mathematician and astronomer.
Wrote Xugu suanjing (Continuation of Ancient Mathematics) of 22 problems. Solved cubic equations by generalization of algorithm for cube root.

Translations of Indian mathematical works.
By 600 C.E., 3 works, since lost. Levensita, Indian astronomer working at State Observatory, translated two more texts, one of which described angle measurement (360 degrees) and a table of sines for angles from 0 to 90 degrees in 24 steps (3 3/4 degree) increments.
Hindu decimal numerals also introduced, but not adopted.

Yi Xing (683-727) tangent table.

Jia Xian (c. 1050)
Written work lost. Streamlined extraction of square and cube roots, extended method to higher-degree roots using binomial coefficients.

Qin Jiushao (c. 1202 - c. 1261)
Shiushu jiuzhang (Mathemtaical Treatise in Nine Sections), 81 problems of applied math similar to the Nine Chapters. Solution of some higher-degree (up to 10th) equations. Systematic treatment of indeterminate simultaneous linear congruences (Chinese remainder theorem). Euclidean algorithm for GCD.

Li Chih (a.k.a. Li Yeh) (1192-1279)
Ceyuan haijing (Sea Mirror of Circle Measurements), 12 chapters, 170 problems on right triangles and circles inscribed within or circumscribed about them. Yigu yanduan (New Steps in Computation), geometric problems solved by algebra.

Yang Hui (fl. c. 1261-1275)
Wrote sevral books. Explains Jiu Xian's methods for solving higher-degree root extractions. Magic squares of order up through 10.

Guo Shoujing (1231-1316).
Shou shi li (Works and Days Calendar). Higher-order differences (i.e., higher-order interpolation).

Zhu Shijie (fl. 1280-1303)
Suan xue qi meng (Introduction to Mathematical Studies), and Siyuan yujian (Precious Mirror of the Four Elements). Solves some higher degree polynomial equations in several unknowns. Sums some finite series including (1) the sum of n^2 and (2) the sum of n(n+1)(n+2)/6. Discusses binomial coefficients. Uses zero digit.

Reference : http://aleph0.clarku.edu/~djoyce/mathhist/china.html